Comfort modelling in semi-outdoor spaces

Indoor comfort modelling is well known and mastered thanks to empirical indices or heat balance equations of the individual. In open buildings, called semi-outdoor spaces, assessing comfort is a considerable effort as rapid variations of ambient conditions require the transient modelling of human metabolism.

Keywords: semi-outdoor spaces, comfort index, transient modelling.

The widespread comfort index “Predicted Mean Vote” (PMV) by (Fanger 1970) gives a prediction of the average mean thermal sensation depending on the steady-state sensible and latent heat load on the individual in his environment. The predicted percentage of dissatisfied (PPD) is linked to the PMV with a Gaussian-like relationship (see Figure 1), depending directly on the heat load that results from the ambient conditions.

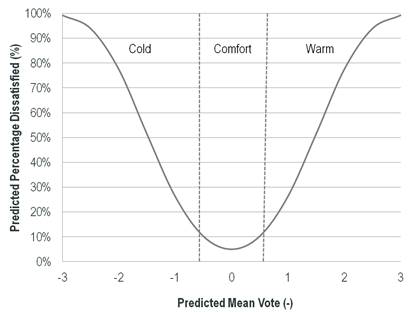
Figure 1. PMV to PPD relationship.

This comfort index has been developed for indoor conditions with wall temperatures that do not deviate much from the air’s one, and for individuals whose metabolism has reached steady-state, thus implying an exposition time to ambient conditions reaching several hours. The PMV can hence no more be applied to the outdoor or semi-outdoor cases, especially due to the rapid variations of air velocities, mean radiant temperatures and because the average time spent in such places (for instance railway stations) is short compared to the human metabolism reaction time. A detailed, transient simulation of heat and vapour transfer is then necessary for this type of spaces.

Decades of research in the fields of comfort and applied medical biology have allowed constructing predictive models of the human thermal behaviour. They depend on ambient conditions and human thermal control reactions of the metabolism.

Comfort in semi-outdoor spaces

The “Standard Effective Temperature” (or “SET*”) is a comfort index originating from the research of (Nishi et Gagge 1977). It is based on the transient modelling of the human metabolism, including the mechanisms of thermoregulation caused by the ambient conditions or the activity level (perspiration, sweating, shivering, vasoconstriction or dilation). It was adapted to outdoor and semi-outdoor spaces by (Pickup et de Dear 2007) and referred to as “Out_SET*”.

The central idea of the SET* is to convert the ambient conditions studied into a single temperature for a reference case: low air velocity (v~0.1 m/s), mean radiant temperature being equal to the air temperature and ambient relative humidity of 50%. In these conditions, the SET* is the operative temperature that leads to the same physiological reactions as the studied ambient conditions, this means: the same skin wetness and skin temperature after a given exposition time (hence SET* or Out_SET* and duration of exposition are inseparable). This little-used approach is however proposed as a reference for the study of thermal comfort by the (ASHRAE 2013).

The inability of steady-state methods to yield a correct prediction of the level of comfort in varying ambient conditions is illustrated on Figure 2: the metabolic evolution of an individual suddenly exposed to a hot summer environment is plotted, starting from the set temperatures of the body. In these conditions, one can observe that the core and skin temperatures do not approach their steady-state values before 30 minutes (“steady” on the figure), whereas the dynamics of regulation via skin wetness are slower and take about ~100 minutes to stabilize. Winter conditions produce an even slower response and body temperatures take 3 to 4 hours to stabilize. Using a steady-state approximation for the estimation of thermal comfort leads to an underestimation of discomfort as far as short expositions are concerned (below one hour), as underlined in (Höppe 2002).

Figure 2. Out_SET* model, comparison between the steady-state and transient physiological values for summer conditions.

These statements are true for the “step response” (as per Figure 2), however they remain valid for changing ambient conditions, especially air velocity and solar flux, both having a strong impact on human heat balance. As an illustration, 800 W/m² of incident solar radiation are equivalent to a 27 K increase of the mean radiant temperature.

However, if the SET*/Out_SET* provides a tool to evaluate an environment, it does not give the designer a comfort scale. The research done by (Int Hout 1990) has allowed to bridge this gap, conciliating the “indoor” PMV and SET* thanks to the reference indoor environment provided in the calculation of the SET*. This allows for a quantitative estimation of the level of comfort equivalent to a given SET* or Out_SET* temperature, using the classical PMV approach, renamed as PMV* in this case.

Influence of air velocities

The influence of air velocity on the comfort zone is shown on Figure 3. One can observe the comfort zone position (−0.5<PMV*<+0.5) on the psychrometric diagram for two velocity magnitudes. When air speed increases, the comfort zone shifts towards higher temperatures: the reduction of the temperature difference is compensated by an increase of the convective heat exchange coefficient, allowing for a stable heat balance even at higher temperatures.

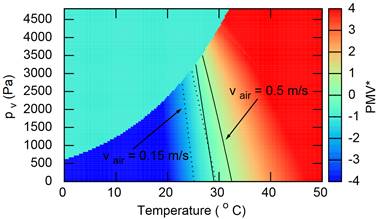
Figure 3. PMV* - Comfort zone position for =0.15 m/s (dotted line) and = 0.5 m/s (solid line).

The heat and vapour transfer resistance properties of clothing are also dependent on the air velocity. Infiltration and “pumping” due to the individuals’ physical activity reduce the insulating properties of cloth in comparison to the still air situation.

A method for evaluating the lessening of heat and vapour transfer resistance properties was provided by (Holmér, et al. 1999) and (Havenith, et al. 1999), which participated in the elaboration of the norm ISO 9920. Based on their study, the influence of air velocity on clothing properties is characterized by a strong reduction of transfer resistance: compared to a still air environment, a 1 m/s air velocity leads to a 40% decrease of convective resistance and a 60% decrease of vapour transfer resistance. Figure 4 shows the position of the comfort zone on the psychrometric chart with and without the modification of cloth properties depending on air velocities. Such a correction leads to a shift of the comfort zone towards higher temperatures, which is equivalent to wearing clothes that provide less insulation.

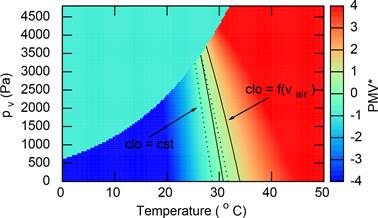
Figure 4. PMV* - Comfort zone position with (solid line) and without (dotted line) correction of cloth insulation.

Conclusion

Given the strong variation of ambient conditions and the short duration of stay, classical indexes are not suited to the estimation of comfort in semi-outdoor spaces, the latter being characterized by highly transient phenomena. It his however possible to qualify comfort rationally, using a refined simulation of the temporal evolution of human metabolism. A detailed knowledge of incident solar fluxes and air velocities is the obvious corollary to such modelling. The calculation of velocity distributions is a challenge in terms of computability, however the rapid evolution of computing capacity and performance, along with the development of affordable on-line ‘cloud’ services make such approaches possible. The metabolic history also has a sensible effect on comfort perception, as mentioned in (Walther et Barry 2016).

References

ASHRAE. Standard 55-Thermal Environmental Conditions for Human Occupancy. 2013.

Fanger, P. O. Thermal Comfort: Analysis and applications in environmental engineering. Mc Graw Hill, 1970.

G. S. Brager, R. de Dear. "A Standard for Natural Ventilation." ASHRAE Journal Volume 42 , n°10, 2000: 21-28.

Havenith, G., I. Holmér, E.A. Den Hartog, and K.C. Parsons. "Clothing Evaporative Heat Resistance - Proposal for Improved Representation in Standards and Models." Ann. Occ. Hyg., 1999: (43-5):339-346.

Holmér, I., H. Nilsson, G. Havenith, and K. Parsons. "Clothing Convective Heat Exchange -Proposal for Improved Prediction in Standards and Models." Annals of Occupational Hygiene, 1999: (43)5-329-337.

Höppe, J. "Different aspects of assessing indoor and outdoor thermal comfort." Energy and Buildings, 2002: 661-665.

Int Hout, D. "Thermal comfort calculations / A computer model." ASHRAE Transactions, 1990: (96)840-844.

Nishi, Y., and A.P. Gagge. "Effective Temperature Scale Useful for Hypo-and Hyperbaric Environments." Aviation, Space, and Environmental Medicine, 1977: 97-107.

Pickup, J., and R. de Dear. "An outdoor thermal comfort index (Out_SET*) - Part I - The model and its assumptions." In Biometeorology and Urban Climatology at the Turn of the Millennium., 279-283. Geneva: WMO, 2007.

Walther, E., and R. Barry. "Réduction des incertitudes de modélisation de ventilation naturelle et prédiction du confort en espace semi-ouvert." IBPSA France. Champs-sur-Marne, 2016.