Natural ventilation uncertainties in building energy simulations

With the increase of building envelope’s performances, wind effects on natural ventilation represent an increasing share in energy consumption. In classical building energy simulations, those effects are extremely simplified in comparison to reality; however numerical simulations can significantly improve the quality of predictions.

Keywords: natural ventilation, pressure coefficient, building energy simulation

Calculation of wind driven natural ventilation in building energy simulations

To illustrate the issue, let us consider the trivial case of a building with wind driven cross-ventilation. The standard Building Energy Simulation (BES) tools compute the transversal cross-ventilation between to openings a and b at a same height z using the Bernoulli equation:

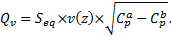
With v(z) is the wind velocity at height z, and Cp is the pressure coefficient on each opposite façades. The effective opening area Seq is a weighted average of each individual opening area as well as their respective discharge coefficient, as in the following equation:

 .

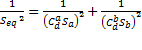Sa and Sb represent the actual surface area of both openings. To overcome Bernoulli hypothesis of non-viscous fluids, their respective discharge coefficients  and  are added. They stand for two distinct phenomena reducing the theoretical flow. On one hand, the flow vein is contracting after the opening due to jets inertial effects. The cross-ventilation is thus reduced by a coefficient Cc, equal to the surface ratio between the jet area after the opening and the actual opening area (an illustration presented in Figure 1, for two simplified openings). On the other hand, the viscous friction also tends to reduce the airflow. It is usually taken into account through a coefficient Cf, usually taken between 0.95 and 0.99.

The discharge coefficient is thus defined by Cc = Cc ´ Cf. The typical value given in the (ASHRAE 1997) standard and several natural ventilation simulation tools (CONTAM, IES-VE MacroFlo, EnergyPlus) ranges from 0.60 to 0.65.

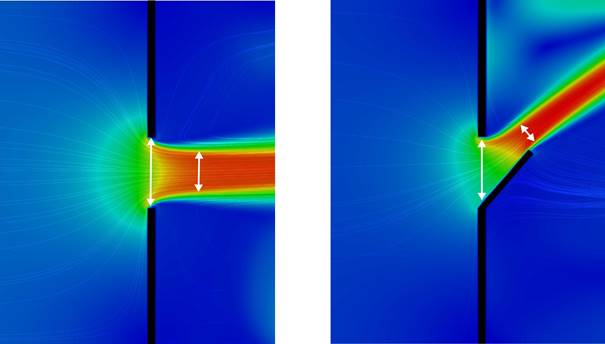
Figure 1. Contraction illustration for two simplified openings.

Since neither the stagnation pressure at the opening height, nor at the actual pressure gap across the two openings a and b are known in classical BES, a pressure coefficient Cp is introduced for each façade. It represents a fraction of the undisturbed flow’s dynamic pressure, and can be either positive or negative, in case of overpressure or depression.

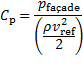
pfaçaderepresents the stagnation pressure, ρ the air density, and vrefthe wind speed at reference height. According to the software accuracy, the Cp coefficient is approximated according the empirical relations, valid only for rectangular buildings, with a shape factor close to one. Sometimes the inflow angle is also taken into account by a corrective factor, as well as the building height influence (Swami et Chandra 1988), (Akins, Peterka et Cermak 1979).

The undisturbed flow velocityvref is taken equal to closest weather station data. To ascertain the wind speed at the opening height z, a logarithmic law describing the atmospheric boundary layer is used:

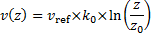
To model the surroundings of the studied building, the profile of the atmospheric boundary layer can be adjusted by the terrain constant, the coefficients ko andzo. They represent respectively the apparent terrain’s roughness and the roughness’ height. ko usually ranges from 0.14 to 0.25, and zo from 0.5 mm to 2 m according to the terrain (sea, lake, snow field, desert, or at the opposite a tropical forest or a dense city center).

Modeling critical review

Reality is often very different from the theory presented above due to buildings complex shapes, exact location within an urban context or the actual shapes of openings. In the following paragraphs, we will demonstrate the possible biases on each modelling parameter.

Discharge coefficient: The Cd coefficient is usually misdocumented by the manufacturers since it relies on many variables. (Salliou 2011) and (Regard 2000) noted that it may vary according to the opening ratio, the temperature difference between the inside and outside or the wind speed. In addition, those authors calculated that this variation ranges from Cd= 0.1 to Cd= 2, in other word from 10% to 200% influence on the flow across the opening. However, it is difficult to lift this uncertainty without a wind tunnel experiment or a numerical simulation. According to the building of interest, the hypothesis on the Cd should be conservative at best, and the results should be properly interpreted.

Pressure coefficient: Those coefficients vary strongly per the wind direction, its magnitude close to façades, building shapes and urban surroundings. Even for simple building geometries, the pressure coefficients are not homogeneous throughout façades. Figure 2 displays a simulation result in terms of Cp, where the values can be contrasted on a unique façade, ranging from slightly negative to positive values in certain areas of a same wall.

Figure 2. Façade pressure coefficient unevenness – Chambéry train station urban environment.

Reference air velocity: This parameter is taken from the closest weather station, for which the exact measurement height is usually unknown, nor the precise location. It is hence often difficult to ascertain precisely the actual wind speed near the location of interest. The velocity around buildings also depends on the topography, the close and distant urban settings with their respective roughness’s. Figures 3 & 4 depicts the flows complexity such areas, in plane and sectional view.

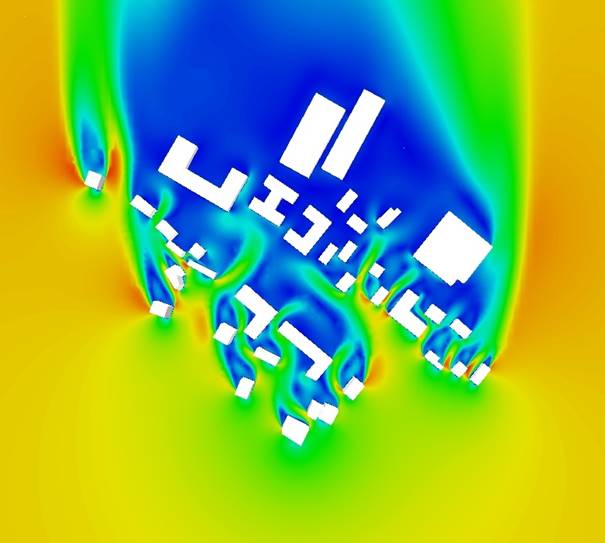
Figure 3. Velocity field fluctuation in urban environments - plane view.

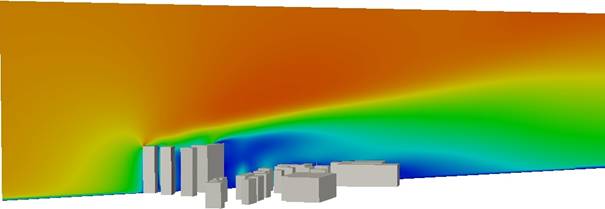
Figure 4. Velocity field fluctuation in urban areas – sectional view.

The uncertain parameters reduction should thus be undertaken using computational fluid dynamics (CFD) simulations. The use of an open-source or purchase-available software that solve the Reynolds-averaged Navier-Stokes equations coupled to a mass-balance model (RANS) is then necessary, using for instance a  k − e turbulence modelling.

This approach allows the explicit determination the Cp on each façade of interest, according to the annual wind data and urban environment. It reduces the near-building velocities and pressure coefficient uncertainties. Those results are then taken as inputs for the annual hourly BES. It should nevertheless be reminded that this approach only considers wind effects: the buoyancy driven ventilation can be evaluated through a Froude’s numbers condition.

References

Akins, R.E., J.A. Peterka, and J.E. Cermak. "Averaged Pressure Coefficients for Rectangular Buildings." Proceedings of the Fifth International Wind Engineering Conference. Fort Collins, 1979. 369-380.

ASHRAE. "Ventilation and Infiltration." In HVAC Fundamentals Handbook, 492-519. Atlanta: ASHRAE Handbook Editor, 1997.

Havenith, G., I. Holmér, E.A. Den Hartog, and K.C. Parsons. "Clothing Evaporative Heat Resistance - Proposal for Improved Representation in Standards and Models." Ann. Occ. Hyg., 1999: (43-5):339-346.

Holmér, I., H. Nilsson, G. Havenith, and K. Parsons. "Clothing Convective Heat Exchange -Proposal for Improved Prediction in Standards and Models." Annals of Occupational Hygiene, 1999: (43)5-329-337.

Regard, Muriel. Contribution à l'étude des mouvements d'air dans le bâtiment à l'aide d'un code de champ. Thèse de doctorat, Lyon: INSA, 2000.

Salliou, Jean-Rémy. Analyse de l'influence de paramètres géométriques et physiques sur le coefficient de décharge appliqué à la ventilation dans le bâtiment. Thèse de Master Recherche, Nantes: Ecole Supérieure d'Architecture de Nantes, 2011.

Swami, M.V., and S. Chandra. "Correlations for pressure distribution on buildings and calculation of natural-ventilation airflow." ASHRAE Transactions, 1988: 243-266.